[HAM] 직교위상 신호(Quadrature Signals)는 복잡하지 않다. 복합적 일 뿐, (5/5)
A Quadrature Signals Tutorial: Complex, But Not Complicated
by Richard Lyons
[ 원본출처: https://dspguru.com/dsp/tutorials/quadrature-signals/ ]
---------------------------------
주) 아래 번역 중 [] 안의 내용은 제가 부연설명 삼아 추가한 것입니다. 틀릴 수 있으니 미리 양해를 구합니다. 오류나 미진한 부분이 있다면 기탄없는 지적 바랍니다. -역자-
---------------------------------
<이전>
A Quadrature-Sampling Example
We can use all that we've learned so far about quadrature signals by exploring the process of quadrature-sampling. Quadrature-sampling is the process of digitizing a continuous (analog) bandpass signal and translating its spectrum to be centered at zero Hz. Let's see how this popular process works by thinking of a continuous bandpass signal, of bandwidth B, centered about a carrier frequency of fc Hz.
지금까지 직교위상 신호에 대해 공부한 것들은 모두 직교위상 샘플링(quadrature-sampling)을 배우기 위한 것이었다. 직교위상 샘플링은 연속된(아날로그 신호를 의미함) 대역통과 신호를 수치화하는 처리다[원문의 bandpass(대역통과)라는 표현은 괴념치 말자. 이세상의 모든 신호라고 하기가 거시기 해서 쓰인 말이다. 관심을 두고있는 신호를 골랐다는 뜻이다.] 그리고 0 헤르츠를 중심에 두고 아날로그 신호를 스펙트럼으로 변환 한다.
[고주파에 실려있던 시간영역의 아날로그 신호는 여러 주파수가 섞여 있었다. '샘플링'이란 이 신호 중 관심을 두고 있는 주파수대의 신호들을 골라 주파수를 바꾸는(translate) 행위다. 전파에 실린 음성신호의 예를 들어보자. 20Hz 에서 3kHz 사이(대역)의 음성 신호를 공중에 전달하기 위해 7Mhz 전자기파(전파)에 실려 있었다면 이 아날로그 신호의 범위는 7.00020 ~ 7.003Mhz가 관심 신호가 된다. 이중 관심 대역은 B 라고 표현된 주파수들이다. B의 범위에 있는 주파수 범위가 7.00020 ~ 7.003Mhz가 되는 것이고. 이 범위 내의 고주파 아날로그 신호를 다시 음성 신호만으로 주파수를 낮추겠다는 뜻이다. 아래 그림 12에의 'original continuous'에서 'desired digitized'로 주파수를 바꾸겠다는 뜻이다. 이때 연속된 값(쉽게 전압치라고 하자)을 수치화 한다. 결국 샘플링의 의미는 주파수도 낮추고 아날로그 신호의 측정치를 숫자화 하겠다는 두가지 의미를 가진다.]
Figure 13. The 'before and after' spectra of a quadrature-sampled signal.
Our goal in quadrature-sampling is to obtain a digitized version of the analog bandpass signal, but we want that digitized signal's discrete spectrum centered about zero Hz, not f_c Hz.
직교위상 샘플링의 목표는 대역통과된 아날로그 신호를 수치화(digitized, 디지털 화) 시키는 것이다. 이때 디지털화 된 신호들의 주파수 분포(spectrum)가 f_c Hz에서 0 Hz 를 중심으로 포진된다.
[그냥 '샘플링'이 아니라 '직교위상 샘플링'이라고 한 이유가 궁금할텐데, 나중에 설명이 되겠지만 직관적으로 살펴보면 샘플링을 두가지 방식('정위상, in-phase'과 '직교위상 quadrature-phase')으로 하겠기에 미리 '직교위상'이란 말을 꺼내 놨다. 그냥 '샘플링'이라고 말하면 '정위상'과 '직교위상'을 구분하지 않았다는 의미이고 '직교위상 샘플링' 이라고 했을 때는 두가지를 모두 일컬어 지칭하는 말이다. 그럼 뭐하러 직교위상 샘플링을 하는지 그 이유를 설명하려는 것이 이 교재의 목적이다.]
That is, we want to mix a time signal with e^(-j2πf_ct) to perform complex down-conversion.
The frequency fs is the digitizer's sampling rate in samples/second. We show replicated spectra at the bottom of Figure 13 just to remind ourselves of this effect when A/D conversion takes place.
샘플링 주파수 fs 는 수치화 장치(digitizer)의 변환율(초당 측정이 이루어지는 횟수)로 샘플/초로 나타낸 숫자다[주파수(frequency)란 초당 몇개, 초당 몇회 등을 의미함]. 그림 13에서 아랫부분의 그래프를 보면 A/D 변환을 수행 한 후 fs 와 -fs 그리고 0Hz 의 세곳에 신호의 복사본이 표시되어 있는 점에 주목해 두자.
OK, ... take a look at the following quadrature-sampling block diagram known as I/Q demodulation (or 'Weaver demodulation' for those folk with experience in communications theory) shown at the top of Figure 14. That arrangement of two sinusoidal oscillators, with their relative 90o phase difference, is often called a quadrature-oscillator.
좋다. 이제부터 직교위상 샘플링을 설명하는 계통도를 봐가면서 I/Q 복조(demodulation)를 알아보기로한다. 통신 이론에 조예가 깊은 사람들 중에는 I/Q 복조를 위버 복조(Weaver demodulation)이라고 부르는 사람도 있다. 직교위상 샘플링의 계통도(block diagram)는 그림 14의 윗 부분에 그려놨다[계통도까지 그려야 한다는 것은 처리과정이 있다는 뜻이다. 그저 반송파 쳐내기용 믹서만으로 복조를 끝내는 것이 아니다.] 서로 위상이 90도 차이나는 두개의 삼각함수 파 발진기가 위치하고 있는데 이 발진기를 직교위상 발진기(quadrature-oscillator) 라고 한다. [cos(2πf_ct + φ)라고 표현된 파형에서 φ = π/2 로 놓으면 sin(2πf_ct) 가 된다. 그러니까 cos(2πf_ct) 와 sin(2πf_ct)는 시작 하는 각도의 위치가 90도 만큼 나는데 이걸 있어보이게 표현하면 '위상차가 φ/2 만큼 차이'라고 했다.]
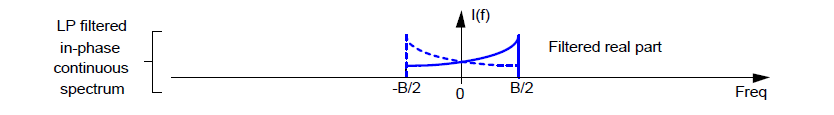
Those e^(j2πfct) and e^(-j2πfct) terms in that busy Figure 14 remind us that the constituent complex exponentials comprising a real cosine duplicates each part of the X_bp(f) spectrum to produce the Xi(f) spectrum. The Figure shows how we get the filtered continuous in-phase portion of our desired complex quadrature signal. By definition, those Xi(f) and I(f) spectra are treated as 'real only'.
----------------------------------------------
[원문이 간결함을 추구 하려 해서 그런지 우리말로 옮기기 곤란하다. 그래서 원문을 근거로 그림 14를 설명한다. 먼저 표기법 부터 살펴보자.
i) 신호의 표기법에 대하여: 신호를 소문자와 대문자로 표기되어 있다. 소문자로 표기된 것 x(t)은 시계열 신호, 대문자 표기 X(f)는 이에 대응하는 주파수 스펙트럼이다. x()의 인자가 t 로 시간이며 X()의 인자 f 는 주파수다. 신호에 첨자를 붙인 것은 처리되었다는 뜻이다. x_bp(t)는 x(t)가 대역 필터를 통과시킨 신호다.
ii) 직교위상 샘플링의 계통도: 계통도까지 그려야 한다는 것은 처리과정이 있다는 뜻이다. 믹서, 저역통과 필터 그리고 A/D 변환까지 끝난후 비로서 샘플링이 완료된다. 두개의 변환절차가 동시에 이뤄지는데 하나는 cos(2πf_ct)와 섞인 경로로 정위상(in-phase) 샘플링이다. 이 경로로 출력되는 디지탈 신호는 i(n) 이다. 다른 하나는 sin(2πf_ct)와 섞인 경로로 직교위상(quadrature phase) 샘플링이다. 출력되는 디지탈 신호는 q(n) 이다.
iii) 이산 신호(discrete signal): 신호를 표기하면서 인자가 t 에서 n 으로 바뀐것을 인지했다면 눈썰미가 아주 좋은 것이다. 시간의 연속적인 신호체계(아날로그)에서 시간 분할된 순서(이것을 '이산'이라고 함)로 측정되어 숫자로 바뀌었다(디지탈 화)는 의미다.
안테나와 공진회로를 거쳐 입력된 전파신호를 x_bp(t)라고 하자. x_bp(t)에는 반송파라고 하는 라디오 주파수 f_c 가 섞여있다.
x_bp(t) 는 실제 신호다. 이 실제 신호는 기본적으로 양측파대(dual-side band)로 존재하는데 주파수 스펙트럼을 분석하여 X_bp(f)를 보면 굵은 실선으로 나타낸 상측파대(uppper side band)와 점선으로 나타낸 하측파대(lower-side band)로 나뉘는 것을 알 수 있다. 상측파대 신호는 양의 주파수 f_c 주변에 포진한다. 하측파대는 음의 주파수 -f_c를 중심으로 포진해 있다. 하측파대 신호들은 상측파대 신호를 0 Hz을 축으로 거울상(mirror image)으로 존재한다. 하측파대 신호를 이미지(image) 신호라고도 한다. 복소수의 허수(imaginary number)와는 다른 뜻이니 혼돈하지 말자.
다운 컨버젼 하기전에 BPF로 원하지 않은 주파수 대역 밖의 신호를 차단 하면,
멀티 밴드의 가변 주파수 발진기(VFO)를 쓰는 무선기기인 경우 밴드마다 BPF를 둘 수 없으므로 중간 주파수로 1차 다운 컨버젼 하여 대역 통과 필터를 통과 시킨후 고정 주파수의 신호로 2차 다운 컨버젼하여 원하는 신호를 얻는다[헤테로다인 라디오의 원리].
그런데 주파수가 음수가 될 수 있을까? 자연계가 돌아가는 사정이 그러하다. 다만 이런 사정을 알아 냈다는 것이 대단할 뿐이다. 어쨌든 이런 사정을 수학적으로 모형화 한 것이 복소 지수함수(complex exponentials)다.
실제 신호 x_bp(t)에서 대역 B 범위의 신호들을 추출하려면 주파수 f_c인 삼각함수 파를 섞어 반송파를 제거하는데 이를 다운-컨버젼이라고 한다. 이 삼각함수 파 역시 실제 신호다. 양의 주파수 f_c와 음의 주파수 -f_c를 가지고 있다.
다운-컨버젼을 위해 발진기에서 주파수가 f_c인 신호를 만들어 섞는다. 이때 주파수 f_c가 동일 하면서 위상이 90도(π/2) 다른 신호 cos(2πf_ct)와 sin(2πf_ct)를 각각 곱한다. 이 곱하기가 믹서다. x_bp(t)와 cos(2πf_ct) 를 섞어 다운 컨버젼한 신호를 '정위상(in-phase)' 신호 x_i(t) 라 하고 sin(2πf_ct) 를 섞어 다운 컨버젼한 신호를 '직교위상(quadrature-phase)' 신호 x_q(t) 라 한다. 왜 나누는지는 차차 알아보기로 하고 실제 코사인과 사인을 복소 지수함수로 나타냈던 그림 9를 상기해두기 바란다.
------------------------------------------------------------------
입력 x_bp(t)가 정위상 신호로 샘플링 되는 과정을 보자. 오일러 공식에서 유도됐던 cos(2πf_ct)는 식 (10)과 같다. x_bp(t)와 곱해보자.
x_bp(t)는 주파수가 합쳐 만든 신호지만 계산 편의를 위해 cos(2πft)로 놨다. 뭐로 놔도 좋은데 실제 신호라는 것만 유의하자. 곱하고 난후 신호의 스펙트럼은 두 주파수의 합과 차의 성분으로 나뉜다. 물론 음의 주파수 쪽으로도 거울상이 있다.

다운 컨버젼이 목적이므로 주파수 합의 대역은 필요 없으니 저역통과 필터(LPF)로 걸러내자. 걸러져 나온 신호 i(t)는 아직 아날로그 신호다. A/D 변환기를 거쳐 숫자화 하여 얻은 신호가 바로 정위상 샘플링(in-phase sampling) 신호 i(n)이다.
Likewise, Figure 15 shows how we get the filtered continuous quadrature phase portion of our complex quadrature signal by mixing x_bp(t) with sin(2πfct).
이번에는 x_bp(t)에 위상이 cos(2πfct)에서 90도 돌아간 sin(2πfct)을 섞어보자. 복소 직교위상 신호의 직교위상 성분을 얻어낸수 있다.
Figure 15. Spectra within the quadrature phase (lower) signal path of the block diagram.
------------------------------------------------------------------
이번에는 입력 x_bp(t)가 직교위상 신호로 샘플링 되는 과정을 보자. 오일러 공식에서 유도됐던 sin(2πf_ct)는 식 (11)과 같다. x_bp(t)와 곱해보자. 앞서 정위상 샘플링에서 x_bp(t)를 cos(2πft)로 놨었는데 같은 조건으로 놓아야 한다. 물론 x_bp(t)도 복소 신호로 표현하면 실수부와 허수부를 가진 신호 일 수 있지만 계산의 편의를 위해 허수부는 없는 신호라고 간주했다.
x_bp(t)를 X_bp(f)로 변환하면 이렇다. 앞서 그림 14와 동일한 신호다.
여기에 sin(2πf_ct) 를 곱해보자. 사인은 오일러 등가공식에서 확장한 식 (11)을 활용한다.
계산하고 나면 전체 결과에 j 가 붙는다(imaginary part). 90도 튼 신호와 주파수 섞기로 생성된 신호는 원신호에서 허수부를 꺼내는 것과 같다. 허수부의 크기 X_q(f)가 음의 값을 가진다. 게다가 음의 주파수 쪽에 이미지는 0Hz을 두고 회전 대칭이다.
이번에도 다운 컨버젼이므로 저역통과 필터를 거친 신호를 A/D 컨버젼 해서 q(n) 신호를 얻는다.
------------------------------------------------------------------
Here's where we're going: I(f) - jQ(f) is the spectrum of a complex replica of our original bandpass signal xbp(t). We show the addition of those two spectra in Figure 16.
Figure 16. Combining the I(f) and Q(f) spectra to obtain the desired 'I(f) - jQ(f)' spectra.
This typical depiction of quadrature-sampling seems like mumbo jumbo until you look at this situation from a three-dimensional standpoint, as in Figure 17, where the -j factor rotates the 'imaginary-only' Q(f) by -90 deg, making it 'real-only'. This -jQ(f) is then added to I(f).
-------------------------
그림 17은 I(f)와 jQ(f)를 각각 실수와 허수 평면에 주파수 축으로 확장한 3차원 그래프로 표현했다. 맨 윗 그림은 실수부 I(f) 다. 두번째 그림은 허수부 Q(f)다. 허수부 Q(f)에 -j 연산자가 붙어 있는데 이를 떼내면 -90도 회전하게 되고(세번째 그림) 마침내 실수부 I(f)와 더해진다(네번째 그림). 점선(이미지 성분)은 상쇄되어 사라지고 실선 부분만 더해진다.
Figure 17. 3-D view of combining the I(f) and Q(f) spectra to obtain the I(f) - jQ(f) spectra.
The complex spectrum at the bottom Figure 18 shows what we wanted, a digitized version of the complex bandpass signal centered about zero Hz.
그림 18은 결국 다운 컨버젼(믹싱)과 디지탈 변환(ADC)로 얻은 i(t) - jq(t)를 스펙트럼 상에서 나타냈다. 이 신호는 0 Hz를 중심으로 포진되어 있다.
Figure 18. The continuous complex signal i(t) - q(t) is digitized to obtain discrete i(n) - jq(n).
Some advantages of this quadrature-sampling scheme are:
직교위상 샘플링으로 얻는 몇가지 장점을 나열해 보자면 다음과 같다.
- Each A/D converter operates at half the sampling rate of standard real-signal sampling,
A/D 변환의 샘플링 율이 실제 신호 샘플링 율보다 절반이면 된다. [나이퀴스트 조건이라면 샘플링 주파수는 원하는 신호의 두배 이상 이어야 한다. 하지만 I/Q 신호로 처리하면 샘플링 주파수와 신호의 주파수가 같아도 된다. 사실 I와 Q를 90도 틀어 놨기 때문에 다운 컨버젼 할때 이미 두배의 주파수로 샘플링 한것과 같다.]
- In many hardware implementations operating at lower clock rates save power.
낮은 주파수에도 넓은 대역의 주파수 샘플링을 할 수 있어서 소모전력을 줄일 수 있다.
- For a given fs sampling rate, we can capture wider-band analog signals.
샘플링 주파수를 가지고 광대역 아날로그 신호를 취득할 수 있다. [위와 같은 얘기]
- Quadrature sequences make FFT processing more efficient due to covering a wider frequency range than when an FFT’s input is a real-valued sequence.
직교위상 샘플링은 FFT를 수월하게 수행한다. [FFT 입력으로 실수부와 허수부를 가진 신호가이어야 한다. I와 Q 로 다운 컨버젼해 놨기 때문에 입력 신호를 복소수화 하기 위해 별도의 절차가 필요없이 곧장 FFT에 들어갈 수 있다.]
- Because quadrature sequences are effectively oversampled by a factor of two, signal squaring operations are possible without the need for upsampling.
직교위상 샘플링으로 이미 두배 속도로 샘플링 한 것이므로 업 샘플링 할 필요 없이 제곱신호를 구할 수 있다.
- Knowing the phase of signals enables coherent processing.
[복소 공간의 신호를 얻은 탓에] 위상 정보를 알고 있으므로 간섭 처리를 수월하게 수행한다.
- Quadrature-sampling makes it easier to measure the instantaneous magnitude and phase of a signal during demodulation.
직교위상 샘플링은 [실수부와 허수부를 가진 복소 신호이므로] 세기와 위상을 가지고 시작하기 때문에 복조가 수월하다.
Returning to the Figure 14 block diagram reminds us of an important characteristic of quadrature signals. We can send an analog quadrature signal to a remote location. To do so we use two coax cables on which the two real i(t) and q(t) signals travel. (To transmit a discrete time-domain quadrature sequence, we'd need two multi-conductor ribbon cables as indicated by Figure 19.)
다시 그림 14로 가보자. 직교위상 신호의 아주 중요한 특성을 알 수 있다. 시계열 신호 i(t)와 q(t)를 원격지에 전송 하려면 섬세한 동축 케이블을 써야 하지만 A/D 변환된 디지탈 신호는 그냥 두줄짜리 전선이면 된다. [아날로그 전송선로에 비해 디지털 전송선로는 훨씬 수월하다. 이는 디지털 통신의 일반적인 장점이다.]
Figure 19. Reiteration of how quadrature signals comprise two real parts.
To appreciate the physical meaning of our discussion here, let's remember that a continuous quadrature signal xc(t) = i(t) + jq(t) is not just a mathematical abstraction. We can generate xc(t) in our laboratory and transmit it to the lab down the hall. All we need is two sinusoidal signal generators, set to the same frequency fo. (However, somehow we have to synchronize those two hardware generators so that their relative phase shift is fixed at 90o.) Next we connect coax cables to the generators' output connectors and run those two cables, labeled 'i(t)' for our cosine signal and 'q(t)' for our sine wave signal, down the hall to their destination.
Now for a two-question pop quiz. In the other lab, what would we see on the screen of an oscilloscope if the continuous i(t) and q(t) signals were connected to the horizontal and vertical input channels, respectively, of the scope? (Remembering, of course, to set the scope's Horizontal Sweep control to the 'External' position.)
Figure 20. Displaying a quadrature signal using an oscilloscope.
Next, what would be seen on the scope's display if the cables were mislabeled and the two signals were inadvertently swapped?
The answer to the first question is that we’d see a bright 'spot' rotating counterclockwise in a circle on the scope's display. If the cables were swapped, we'd see another circle, but this time it would be orbiting in a clockwise direction. This would be a neat little demonstration if we set the signal generators' fo frequencies to, say, 1 Hz.
This oscilloscope example helps us answer the important question, "When we work with quadrature signals, how is the j-operator implemented in hardware?” The answer is we can’t go to Radio Shack and buy a j-operator and solder it to a circuit board. The j-operator is implemented by how we treat the two signals relative to each other. We have to treat them orthogonally such that the in-phase i(t) signal represents an East-West value, and the quadrature phase q(t) signal represents an orthogonal North-South value. (By orthogonal, I mean that the North-South direction is oriented exactly 90o relative to the East-West direction.) So in our oscilloscope example the j-operator is implemented merely by how the connections are made to the scope. The in-phase i(t) signal controls horizontal deflection and the quadrature phase q(t) signal controls vertical deflection. The result is a two-dimensional quadrature signal represented by the instantaneous position of the dot on the scope's display.
A person in the lab down the hall who's receiving, say, the discrete sequences i(n) and q(n) has the ability to control the orientation of the final complex spectra by adding or subtracting the jq(n) sequence as shown in Figure 21.
Figure 21. Using the sign of q(n) to control spectral orientation.
The top path in Figure 21 is equivalent to multiplying the original xbp(t) by e^(-j2fct), and the bottom path is equivalent to multiplying the xbp(t) by e^(j2fct). Therefore, had the quadrature portion of our quadrature-oscillator at the top of Figure 14 been negative, -sin(2fct), the resultant complex spectra would be flipped (about 0 Hz) from those spectra shown in Figure 21.
While we’re thinking about flipping complex spectra, let’s remind ourselves that there are two simple ways to reverse (invert) an x(n) = i(n) + jq(n) sequence’s spectral magnitude. As shown in Figure 21, we can perform conjugation to obtain an x'(n) = i(n) - jq(n) with an inverted magnitude spectrum. The second method is to swap x(n)’s individual i(n) and q(n) sample values to create a new sequence y(n) = q(n) + ji(n) whose spectral magnitude is inverted from x(n)’s spectral magnitude. (Note, while x'(n)’s and y(n)’s spectral magnitudes are equal, their spectral phases are not equal.)
Conclusions
결론
This ends our little quadrature signals tutorial. We learned that using the complex plane to visualize the mathematical descriptions of complex numbers enabled us to see how quadrature and real signals are related. We saw how three-dimensional frequency-domain depictions help us understand how quadrature signals are generated, translated in frequency, combined, and separated. Finally we reviewed an example of quadrature-sampling and two schemes for inverting the spectrum of a quadrature sequence.
이렇게 직교위상 샘플링에 대한 짧은 교육을 마친다. 복소수 평면에 복소신호의 수학적인 의미를 그려보고 복소신호가 실신호에 어떻게 관련지을 수 있는지 살펴봤다. 직교위상 신호를 어떻게 만들 수 있으며 주파수 영역으로 변환되고 분리된 후 다시 결합 되는 과정을 3차원 주파수 영역 그림을 통해 살펴봤다. 끝으로 직교위상 샘플링과 두 직교위상 신호열의 스펙트럼 뒤집힘 특성에 대해서도 알아봤다[직교위상 샘플링의 장점 중 허수부 신호가 별 처리 없이도 자연스럽세 사라짐. 샘플링 주파수 두배의 대역 신호 처리 가능 함.]
References
[1] D. Struik, A Concise History of Mathematics, Dover Publications, NY, 1967.
[2] D. Bergamini, Mathematics, Life Science Library, Time Inc., New York, 1963.
[3] N. Boutin, "Complex Signals," RF Design, December 1989.
Answer to trivia question just following Eq. (5) is: The scarecrow in The Wizard of Oz.
Have you heard this little story?
While in Berlin, Leonhard Euler was often involved in philosophical debates, especially with Voltaire. Unfortunately, Euler's philosophical ability was limited and he often blundered to the amusement of all involved. However, when he returned to Russia, he got his revenge. Catherine the Great had invited to her court the famous French philosopher Diderot, who to the chagrin of the czarina, attempted to convert her subjects to atheism. She asked Euler to quiet him. One day in the court, the French philosopher, who had no mathematical knowledge, was informed that someone had a mathematical proof of the existence of God. He asked to hear it. Euler then stepped forward and stated:
"Sir, a + bnn = x, hence God exists; reply!"
Diderot had no idea what Euler was talking about. However, he did understand the chorus of laughter that followed and soon after returned to France.
Although it's a cute story, serious math historians don't believe it. They know that Diderot did have some mathematical knowledge and they just can’t imagine Euler clowning around in that way.
----------------------------------
<끝> <처음>




















댓글 없음:
댓글 쓰기